Equations Explained: A Comprehensive Guide To Solving
Can a simple equation hold the key to unlocking complex realities? Indeed, the world of mathematics is a vast and intricate landscape, where seemingly straightforward equations can reveal profound insights into the nature of our universe.
At the heart of this exploration lies the concept of equations mathematical statements that express the equality between two expressions. These equations come in various forms, each with its own unique characteristics and methods of solution. Understanding these different types of equations is crucial for anyone seeking to navigate the intricacies of mathematics and its applications in the real world.
Let's delve into some fundamental equation types and explore their significance.
- Alternatives To Movierulz Stream Movies Safely Legally
- Unveiling Aagmal The Future Of Tech Digital Innovation
Quadratic Equations
A quadratic equation stands as a cornerstone in algebra, characterized by its distinct form: ax + bx + c = 0, where 'a' is not zero. The name "quadratic" comes from "quad," meaning square, due to the presence of a squared variable (x). These equations, representing parabolas when graphed, are vital for modeling various phenomena, from projectile motion to financial models. Solving a quadratic equation typically involves methods like factoring, completing the square, or employing the quadratic formula, a robust tool for finding the roots (or solutions) of any quadratic equation. These roots reveal critical information about the equation's behavior and are fundamental for understanding its applications.
Linear Equations
- Crazyjamjam Nudes Leaks Latest Photos Videos Explore Now
- Discover Your Guide To Hdhub Movies Shows Latest
In contrast to quadratics, linear equations present a different perspective on mathematical relationships. A linear equation is an equation for a straight line. These equations, when graphed, create straight lines, hence the name. The general form of a linear equation is typically expressed as y = mx + c, where 'm' represents the slope and 'c' is the y-intercept. Linear equations are prevalent in numerous fields, including economics (supply and demand), physics (motion), and computer graphics. Their simplicity allows for easy analysis and interpretation, providing insights into the relationship between variables.
For instance, consider the equation y = 2x + 1. The graph of this equation is a straight line. This type of equation involves both x and y, representing the relationship between two variables. If you choose a value for x, you can find the corresponding value for y. For example, when x is 1, y = 2 1 + 1 = 3. So when x = 1, we have y = 3. You can verify that x = 1 and y = 3 lies on the line.
Or, let's say x = 7, y = 2 7 + 1 = 15. So when x = 7, we have y = 15. This shows the consistent relationship between x and y within this linear equation.
Differential Equations
Differential equations introduce a dynamic element, dealing with the rates of change of functions. These equations involve an unknown function, often represented as y = f(x), and one or more of its derivatives. A derivative represents the instantaneous rate of change of a function at a given point. Differential equations are therefore invaluable for modeling dynamic systems in physics, engineering, biology, and finance.
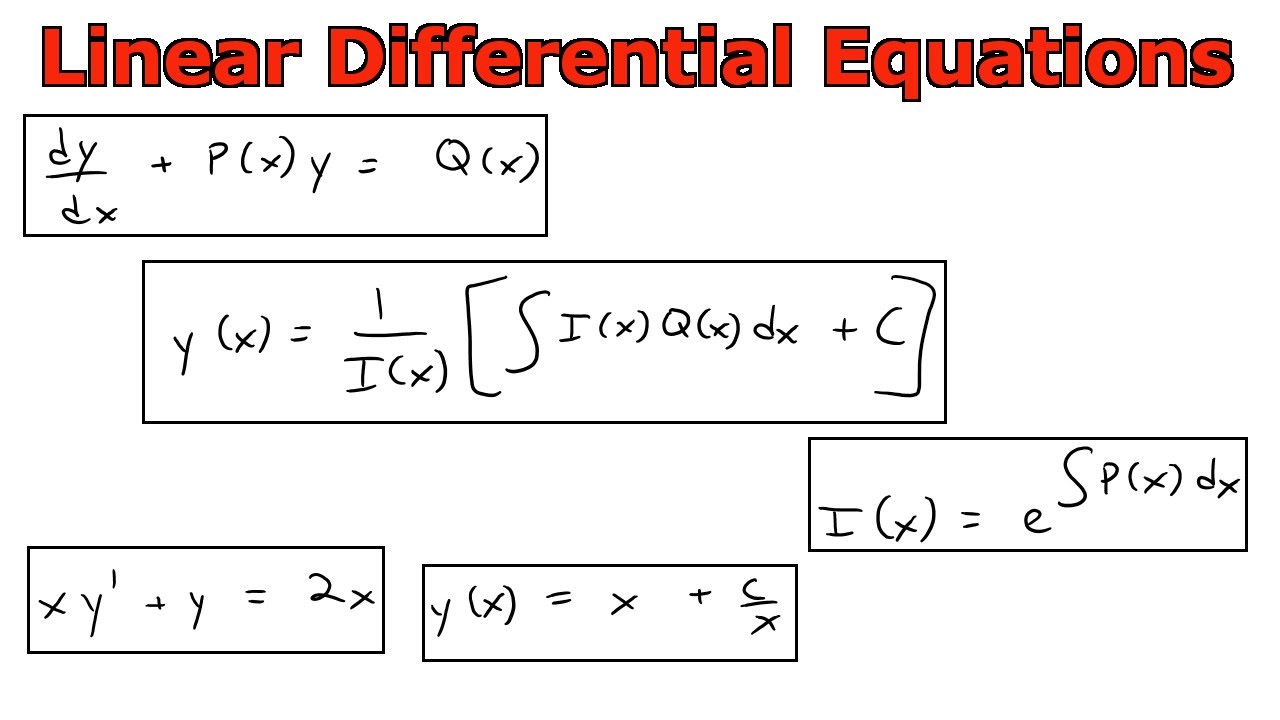
One type of differential equation is a linear first-order differential equation. A first-order differential equation is linear when it can be made to look like this: dy/dx + p(x)y = q(x) where p(x) and q(x) are functions of x. To solve it, a special method can be employed where two new functions of x, u and v, are introduced, and y = uv is proposed. Solving for u and then v allows us to find the solution. Another type is the Bernoulli differential equation, which can be expressed in the standard form: dy/dx + p(x)y = q(x)y^n, where n 1 (making the equation nonlinear).
A solution to a differential equation is a function, y = f(x), that satisfies the equation when the function and its derivatives are substituted into the equation.
Substitution Techniques
To solve some equations, especially differential equations, substitution is a key technique. This technique involves replacing parts of the equation with new variables to simplify the problem. By doing this, the original equation is transformed into a form that is easier to solve. After the solution is found in terms of the new variables, the substitution is reversed to find the solution in terms of the original variables. This approach is particularly useful for solving differential equations that don't fit standard solution methods.
General Form and Standard Form
Sometimes, we encounter equations in general form. For instance, consider an equation in general form: x + y + ax + by + c = 0. The goal is to get it into standard form, often expressed as (x - a) + (y - b) = r. The method to achieve this is "completing the square," applied twice once for 'x' and once for 'y'. This process converts the general equation into a form that reveals the key properties of the geometric shape it represents, often a circle.
Example of Solving an Equation
Consider the equation I(x, y) = 2y - xy + 3y + g(x) (equation 1). Lets differentiate I(x, y) with respect to x and set that equal to m: I/x = m(x, y) = 0 - 2xy + 0 + g'(x) = 3x - 2xy + 2. Simplifying, we have -2xy + g'(x) = 3x - 2xy + 2. This leads to g'(x) = 3x + 2. Integrating g'(x) gives us g(x) = x + 2x + c (equation 2). Now, we can replace g(x) in equation 1 with the result from equation 2.
These are just a few examples of the many types of equations encountered in mathematics. Each one plays a vital role in different fields, helping us to model, understand, and predict various phenomena in the world around us. From the static relationships of linear equations to the dynamic changes described by differential equations, the study of equations offers a powerful lens through which to view and interpret the complexities of reality.
Solving equations and understanding their different forms provides a crucial foundation for anyone wanting to delve deeper into the realms of mathematics, physics, engineering, economics, and various other disciplines.
As we explore these equations, we gain insight into the beauty and power of mathematics a language that allows us to describe, analyze, and ultimately understand the world.
| Category | Details |
|---|---|
| Equation Type | Quadratic, Linear, Differential |
| Quadratic Form | ax + bx + c = 0 (where a 0) |
| Linear Form | y = mx + c |
| Differential Equation Form | dy/dx + p(x)y = q(x) |
| Bernoulli Equation Form | dy/dx + p(x)y = q(x)y^n, where n 1 |
| Solution Methods | Factoring, completing the square, quadratic formula (for quadratics), substitution |
| Applications | Physics, engineering, economics, computer graphics, dynamic modeling |
| Key Concepts | Roots, slope, y-intercept, derivatives, rate of change |
| General to Standard Form | Completing the square |
| Reference | Wikipedia: Equation |
- Polyprincessriri Trending Videos Style Insights You Need
- Explore Sotwe Turk Ifsa Latest Videos Trends Discover Now
Linear Differential Equations YouTube
Exponential Equations With Powers of X YouTube
![[Class 12] Find the general solution of differential equation (x^2+yz](https://d1avenlh0i1xmr.cloudfront.net/c2741cd9-5634-42b8-bd33-6e936b07424c/slide44.jpg)
[Class 12] Find the general solution of differential equation (x^2+yz